
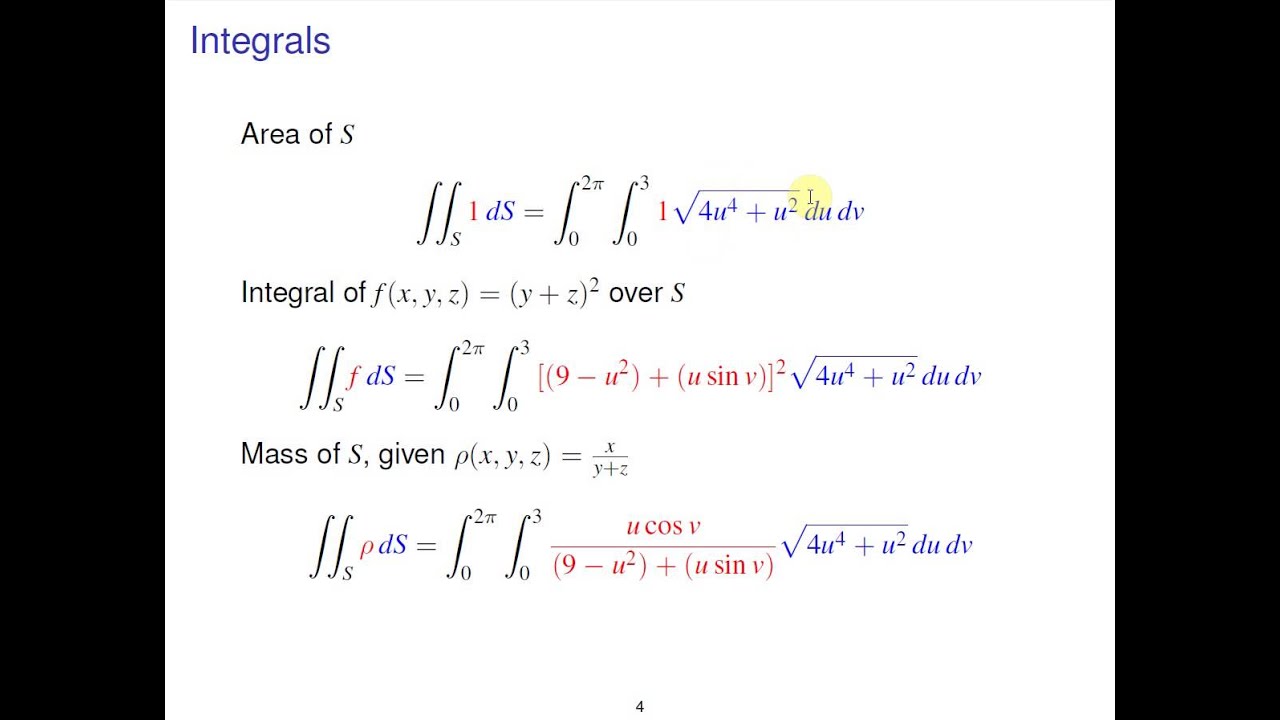
If I = ∫ f(x) dx, where x = g(t) so that dx/dt = g'(t), then we write dx = g'(t) When a function is a function of another function, then we apply the integration formula for substitution. Thus ∫ xe x dx = x ∫e x dx - ∫( 1 ∫e x dx) dx+ c Thus we apply the appropriate integration formula and evaluate the integral. ∫ f(x) g(x) dx = f(x) ∫g(x) dx - ∫ (∫f'(x) g(x) dx) dx + Cįor example: ∫ xe x dx is of the form ∫ f(x) g(x) dx. The integration formula while using partial integration is given as:
When the given function is a product of two functions, we apply this integration by parts formula or partial integration and evaluate the integral. They can be remembered as integration formulas. There are 3 types of integration methods and each method is applied with its own unique techniques involved in finding the integrals. Integration Formulas of Inverse Trigonometric Functions Integration Formulas of Trigonometric Functions Let's move further and learn about integration formulas used in the integration techniques. This inverse process of differentiation is called integration. If the values of functions are known in I, then we can determine the function f. If we differentiate a function f in an interval I, then we get a family of functions in I. These integration formulas are used to find the antiderivative of a function. The integration of functions results in the original functions for which the derivatives were obtained. Integration formulas can be applied for the integration of algebraic expressions, trigonometric ratios, inverse trigonometric functions, and logarithmic and exponential functions.


 0 kommentar(er)
0 kommentar(er)
